Arc Measure vs Arc Length  

In geometry, an arc is an often found, useful figure. Generally, the term arc is used to refer to any smooth curve. The length along the curve from the starting to end point is known as the arc length.

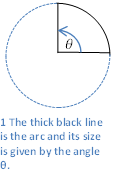
Specifically, the term arc is used for a portion of a circle along its circumference. The size of the arc is usually given by the size of the angle subtended by the arc at the center or the length of the arc. The angle subtended at the center is also known as the angle measure of an arc or informally the arc measure. It is measured in degrees or radians.

The length of the arc differs from the size of the arc, where the length is dependent on the radius of the curve and the angle measure of the arc. This relation between the arc length and the arc measure can be explicitly expressed by the mathematical formula,

S = rθ

where S is the arc length, r is the radius and θ is the angle measure of the arc in radians (this is a direct result from the definition of the radian). From this relation, the formula for the perimeter of a circle or the circumference can be easily obtained. Since the perimeter of a circle is the arc length with an angle measure of 2π radians, the circumference is,

C = 2πr

These formulas are important at every level of mathematics, and many applications can be derived based on these simple ideas. In fact, the definition of the radian is based on the above formula.

When the term arc refers to a curved line, other than a circular line, advanced calculus has to be employed to calculate the arc length. The definite integral of the function describing the path of the curve between two points in space gives the arc length.

What is the difference between Arc Measure and Arc Length? • The size of an arc is measured by the length of the arc or the angle measure of the arc (arc measure). Arc length is the length along the curve while the angle measure of the arc is the angle subtended at the center by an arc. • The arc length is measured in units of length while the angle of measure is measured in units of angles. • The relation between the arc length and the angle measure of the arc is given by S = rθ.

ncG1vNJzZmivp6x7pbXFn5yrnZ6YsqOx07CcnqZemLyue8OinZ%2Bdopq7pLGMm5ytr5Wau26t0Zxkpp2RqMKzsYyapZ1lpqh6or7CZqOeppeptXA%3D